Introduction
Everything we have learned up to this point can be classified as ‘Traditional’ or ‘Standard’ or ‘Tonal’ Jazz Theory. In this module we will be learning some ‘Modern’ Jazz Theory – which involves the gradual rejection of ‘Standard’ Jazz Theory. But first, let’s do a (not so) quick high level ‘Standard’ Jazz Theory Overview to explain how it all fits together. Naturally, this will require me to make some generalisations, so apologies in advance, but here we go.
Jazz Theory Overview
Fundamentals
- There are 12 notes in an octave
- The distance between two notes is called an interval
- Don’t forget this. All music theory can be broken back down into notes and intervals
Intervals
Axiom #1: There are two types of intervals:
- Dissonant; and
- Consonant
The reason some intervals sound consonant and others dissonant has to do with the Overtone Series, but that’s beyond the scope of this lesson.
Axiom #2a: Dissonant intervals create ‘tension’ which sound like they want to ‘resolve’ to consonant intervals
Scales
- A scale is simply some subset of these 12 notes
- There are literally 1,000’s of possible scales
- For historical reasons, the most commonly used scale is the Major Scale – which has 7 notes
- Scales have two components:
- The notes they are comprised of; and
- Their Tonal Centre (root note)
- The Tonal Centre – is the note that feels completely ‘resolved’ and ‘at rest’
- A Tonal Centre can be established in a number of difference ways:
- First/last note
- Lowest/highest note
- Loudest note
- Repeated note
- Once you’ve established a particular tonal centre, all other notes are heard in relation to that tonal centre – including both notes inside and outside the scale
- And each of the 12 notes creates a certain level of tension/dissonance through the implied interval with that tonal centre – and therefore creates some tendency to resolve to that tonal centre (Remember Axiom #2a)
Modes
- If you keep all the notes in the scale the same but change the tonal centre – you create a new ‘Mode’
- C Major = A natural minor
- C Ionian = D Dorian = E Phrygian = etc.
- Modes are just a different way of thinking about music and keys. They allow us to allocate every single chord a particular scale, which is a useful way of thinking about music, especially Jazz music
The Chord-Scale System
- Scales and Chords are interrelated. They are two sides of the same coin
- A scale is a horizontal representation of a particular collection of notes
- A chord is a vertical representation of that same collection of notes
- Scales are built in 2nds
- While chords are built in 3rds
| Scale Name | Scale Notes | Chord Notes | Chord Name |
|---|---|---|---|
| C Ionian (C Major) | C D E F G A B | C E G B D F A | CMaj13 |
| C Mixolydian (F Major) | C D E F G A B♭ | C E G B♭ D F A | C13 |
| C Lydian (G Major) | C D E F# G A B | C E G B D F# A | CMaj13#11 |
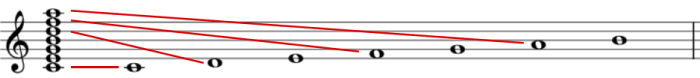
- So in a sense, a scale is a chord. Or more specifically, a chord extended out to the 13th (13th Chord) is its own diatonic scale.
- C Major Scale = CMaj13 chord
- C Mixolydian Scale = C13 chord
- C Lydian Scale = CMaj13#11 chord
- These are the scales you would use to improvise over these chords
- Note: The degrees are based off the Major Scale, which just repeat an octave higher. And any alterations to these degrees are alterations to the notes of the Major Scale
| Deg | 1 | ♭2 = ♭9 | 2 = 9 | #2 = #9 | 3 | 4 = 11 | #4 = #11 | 5 | ♭6 = ♭13 | 6 = 13 | #6 = #13 | 7 |
| CΔ7 | C | D♭ | D | D# | E | F | F# | G | A♭ | A | A# | B |
| Deg | 1 | ♭2 = ♭9 | 2 = 9 | ♭3 | ♭4 = ♭11 | 4 = 11 | #4 = #11 | 5 | ♭6 = ♭13 | 6 = 13 | ♭7 | 7 |
| C-7 | C | D♭ | D | E♭ | F♭ | F | F# | G | A♭ | A | B♭ | B |
- In theory, you play every degree up to the degree listed taking any alterations into account
| Chord | Notes |
|---|---|
| C13 | C E G B♭ D F A |
| C9 | C E G B♭ D |
| C7 | C E G B♭ |
| C13♭9#11 | C E G B♭ D♭ F# A |
- This is different in practice, but I’ll get to that in a moment
Keys
- Just like you can keep all the notes in a scale the same but change the tonal centre to create a new ‘mode’; you can also keep all the notes in a chord the same, but change the ‘root note’ and create a new chord:
| Chord | Notes |
|---|---|
| CMaj13 | C E G B D F A |
| Dm13 | D F A C E G B |
| Em11♭9♭13 | E G B D F A C |
| FMaj13#11 | F A C E G B D |
| G13 | G B D F A C E |
| Am11♭13 | A C E G B D F |
| Bm11♭5♭9♭13 | B D F A C E G |
- Because all these chords all use only the notes from the C Major Scale – that is, all the white notes – these chords are all in the key of C Major, and you can use the C Major scale to improvise over all of them.
7th Chords
- Even though a chord can be extended all the way out to the 13th, in Jazz the basic chord is the 7th chord, which is just the first 4 notes
- There are 4 basic 7th chords (see below)
- There are also diminished and augmented chords, but these work a bit differently
- Each 7th chord has a particular ‘feel’ or ’sound’
| Chord | Notes | Feel |
|---|---|---|
| CMaj7 | C E G B | Happy/Calm |
| C7 | C E G B♭ | Tense |
| Cm7 | C E♭ G B♭ | Sad |
| CmMaj7 | C E♭ G B | Confused Sadness |
- Each of the degrees in a chord can be categorised and analysed
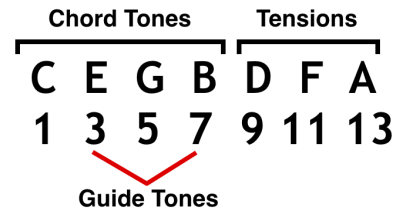
| Degree | Category | Establishes | Altered? |
|---|---|---|---|
| 1 | Chord Tone | Tonality | No |
| 3 & 7 | Guide Tone | Quality | No |
| 5 | Chord Tone | Dim/Aug | Yes (Generally) |
| 9, 11, 13 | Tensions | Adds Colour | Yes |
- 1, 3, 5, 7 = Chord Tones
- 3, 7 = Guide Tones
- 9, 11, 13 = Tensions
- 1 establishes tonality – CMaj, DMaj
- So you can’t alter (flatten or sharpen) the root of a chord without changing the type of chord that it is
- 3, 7 establish quality of the chord – CMaj, Cm, C7
- So again you can’t alter the 3 or 7 of a chord without changing the type of chord that it is
- 5 establishes whether the chord is diminished or augmented
- Generally, can alter the 5 without affecting the chord too much
- 9, 11, 13 just add colour to the chord
- So you can alter these without affecting the type of chord that you are playing
- By that I mean a:
- C13 = C7
- C13♭9#11 = C7
- C7♭5 or C7#5 = is still (generally) a C7
- Cm7 or CMaj7 ≠ C7
- C#7 ≠ C7
- So you CAN alter 5, 9, 11, 13 – without changing the chord type
- But you CANNOT alter the 1, 3, 7 – without changing the chord type
- The Guide Tones (3, 7) are the most harmonically important notes in a chord because they establish the quality
- 1, 5 are less important
- 9, 11, 13 are the least important
- Just like each of the 12 notes creates a differing level of tension against the tonal centre in a scale; each of the 12 notes also creates a differing level of tension against a particular chord, and these can also be categorised:
Non-Dominant Chords
| Category | Degrees | Tension | From CMaj7 |
|---|---|---|---|
| Chord Tone | 1, 3, 5, 7 | Very Consonant/ High Resolution | C E G B |
| Avoid Note | Chord Tone + 1 Semitone | Very Dissonant/ High Tension | D♭ F A♭ |
| Available Tension | Chord Tone + 2 Semitones | Slightly Consonant/ Low Resolution | D F# A |
| Other | All Other Notes | Slightly Dissonant/ Low Tension | E♭ B♭ |
Dominant Chords
| Category | Degrees | Tension | From C7 |
|---|---|---|---|
| Chord Tone | 1 & 5 | Very Consonant/ High Resolution | C G |
| Guide Tones | 3 & ♭7 | Very Consonant/ High Resolution | E B♭ |
| Avoid Note | Guide Tone + 1 Semitones | Very Dissonant/ High Tension | F B |
| Available Tension | All Other Notes | Slightly Dissonant/ Low Tension | D♭ D D# F# A♭ A |
- When building a chord, you can only use
- Chord tones – obviously, as they make up the actual 7th chord; and
- Available tensions – as these retain the general ‘feel’ of the chord.
- So a CMaj9 (which is an available tension) still ‘feels’ like a CMaj7 – it still feels ‘happy’
- Whereas Avoid Notes do not retain the same ‘feel’.
- So a CMaj7♭9 no longer ‘feels’ the same as a CMaj7 – it no longer feels ‘happy’
- A CMaj13, for example, exists in theory but not in practice because the natural 11 (F) is 1 semitone above a chord tone (E) and therefore an Avoid Note.
| Chord | Notes | Avoid Note |
|---|---|---|
| CMaj13 | C E G B D F A | F is an ‘avoid note’ |
- Whereas a CMaj13#11 (C E G B♭ D F# A) exists in practice because it has an F# which is 2 semitones above a chord tone, and therefore an Available Tension
- Now, CMaj13 chords do actually exist in practice, and I’ll explain why in a moment
- Now, when you’re improvising:
- If you play or target the chord tones, especially the guide tones, it will sound very pleasant and consonant and ‘resolved’
- Whereas if you play an avoid note it will sound very dissonant and create a lot of tension which will then sound like it wants to resolve (usually to a chord tone)
- And if you play an available tension, it will sound somewhat consonant and resolved but not as much as a on a chord tone. But again, more on this later.
Chord Ambiguity
- Because not all notes in a chord are of equal importance, it is possible to:
- Omit certain notes (to create shell chords); and
- Substitute chords
- And because of this, chords become a little bit ambiguous
Shell Chords
- It’s possible to retain the ‘feel’ of a chord without playing all the notes in the chord
- Most important notes in a chord are the guide tones (the 3 & 7), again, because they establish the quality of the chord. So just playing these two notes creates the general ‘feel’ of the chord.
| Chord | Notes | Feel |
|---|---|---|
| CMaj7 | E B | Happy/Calm |
| C7 | E B♭ | Tense |
| Cm7 | E♭ B♭ | Sad |
| CmMaj7 | E♭ B | Confused Sadness |
- This, of course, depends on context and on the surrounding chords in the chord progression, but more on that in a moment.
- For this reason, when you come across a CMaj13 or C13 chord, the natural 11 – which is an avoid note – is generally omitted. So a CMaj13 does exist in practice, it just implicitly omits the 11.
| Chord | Notes | Omission |
|---|---|---|
| CMaj13 | C E G B D ( ) A | F is omitted |
Substitute Chords
- For this same reason chords can be substituted. If the substitute chord contains the 3 and 7 of the substituted chord, the two chords will have a similar ‘feel’ and so can act as viable substitutes for each other – for example:
| Chord | Substitute Chord | Substitute Chord Notes |
|---|---|---|
| CMaj7 | Em7 | E G B D |
| C6 | Am7 | A C E G (6th rather than 7th) |
| G7 | D♭7 | D♭ F A♭ B |
| G7 | Bo7 | B D F A♭ |
| Cm7 | E♭Maj7 | E♭ G B♭ D |
Disguised Chords
- But ‘substituting’ chords and ‘omitting’ notes are really the same thing – to use the cliché again – they are two sides of the same coin.
- We can omit any note other than the 3 & 7 without affecting the ‘feel’ of the chord. This means we can omit the root.
- So then if we take a CMaj9 (which is an extended CMaj7) and drop the root note, we get an Em7 (which is a substitute for CMaj7). A Em7 = rootless CMaj9. So a sad chord also sounds like a happy chord – which is curious…
| Chord | Notes |
|---|---|
| CMaj7 | C E G B |
| CMaj9 | C E G B D |
| Rootless CMaj9 | E G B D |
| Em7 | E G B D |
- For this reason chords are very ambiguous and depend on root notes and context (that is, they depend on which chords come before and after it). For example, the notes E G A C could be a C6, Am7 or rootless FMaj7. The only way to tell the difference is by looking at the chord progression and at the root note.
- The most common chord progression that establishes a ‘tonic chord’ is a V-I cadence. So if we have a:
| Chord | Notes | V - I |
|---|---|---|
| C6 | C E G A | G7 | C6 |
| Am7 | A C E G | E7 | Am7 |
| Rootless FMaj9 | ( ) A C E G | C7 | FMaj7 |
Functionality
- The ‘feel’ of a chord also determines it’s ‘function’
- To discuss ‘functionality’ we need to discuss another dissonant interval – the ‘tritone’ (which literally means 3 tones)
- There is only 1 tritone which you can create from the Major Scale – between 4 & 7. This is called the diatonic tritone.
- The Diatonic Tritone dictates something called the ‘function’ of a chord.
- If we stick to the key of C Major, the diatonic tritone is between the F and the B. Each chord in the key of C Major can be categorised into one of three ‘functions’:
| Function | Diatonic Tritone | Chords | Tension |
|---|---|---|---|
| Tonic | No F | - CMaj7 - Em7 - Am7 | Resolution |
| Dominant | F & B | - G7 - Bm7♭5 | High Tension |
| Pre-Dominant | Only F | - Dm7 - FMaj7 | Low Tension |
Remember:
Axiom #2a: Dissonant intervals create ‘tension’ which sound like they want to ‘resolve’ to consonant intervals
- So the diatonic tritone in a V7 (G7) chord wants to resolve to the Major 3rd interval in the Tonic Chord (CMaj7). This creates a V-I perfect cadence and establishes the I chord as the tonic chord. This is the very basis of tonality and of functionality.
- In the same way that you establish the ‘tonal centre’ in a scale, you can also establish a ‘tonic chord’ by using this V-I relationship. For example:
| Em7 | A7 | Dm7 | G7 | ??? |
- This sounds unfinished and unresolved, because we are expecting a CMaj7 chord at the end. Without it, this progression sounds unfinished, so CMaj7 is the tonic chord.
- In the same way that the ‘Tonal Centre’ of a scale feels ‘resolved’ when you’re playing a scale – the ‘Tonic Chord’ also feels ‘resolved’ when you’re playing a chord progression.
- We can extend Axiom #2a a little further to create Axiom #2b (there was always gonna be a 2b) this states that:
Axiom #2b: Slightly dissonant intervals (and chords) want to move to very dissonant intervals (and chords) which want to resolve to consonant intervals (and chords)
- This means that generally a Pre-Dominant chord wants to move to a Dominant chord which wants to move to a Tonic chord
- This brings us to the basis of most traditional tonal music in existence. A typical tonal chord progression would be a:
- II-V-I (Dm7 G7 CMaj7)
- this one of the most common Chord Progressions in Jazz
- Pre-Dominant feels like it wants to move to Dominant which itself feels like it wants to move to Tonic
- II-V-I (Dm7 G7 CMaj7)
- This also depends on context:
| Pre-Dominant | Dominant | Tonic |
|---|---|---|
| Dm7 | G7 | CMaj7 |
| Dm7♭5 | G7 | CMaj7 |
| FMaj7 | Bm7♭5 | CMaj7 |
- The m7♭5 can be either a Pre-Dominant functioning chord or a Dominant functioning chord depending on context (i.e. the surrounding chords).
- This functional movement (from Pre-dominant to Dominant to Tonic) exists because of the diatonic tritone, functionality and Axiom #2b. But also because of something called voice leading.
Voice Leading
- So there are two aspects to Chord Progressions
- Chord itself; and
- Transition to the next chord
- And this transition to the next chord – or through the chord progression is affected by voice leading.
- Let’s take a II-V-I in the key of C Major.
| Dm7 | G7 | CMaj7 |
|---|---|---|
| D | G | C |
| F | B | E |
| A | D | G |
| C | F | B |
- Notice that when each chord transitions to the next:
- 2 notes stay the same (these are called common tones) and;
- 2 notes move by 1 step
- This brings us to Axiom 3
Axiom #3: When moving between chords, the more common tones you have and the smaller the movement between non-common tones, the smoother the chord movement will be
This is the reason the Circle Progression sounds so smooth and is so often used in Jazz Standards.
Scale Ambiguity
- Now, remember that a 13th Chord has all the same notes as its diatonic scale – so you know exactly what scale to use when improvising over that chord
- Well 7th chords are a bit more ambiguous because they are missing a few notes. Two scales I gave earlier were:
| Scale | Scale Notes | Chord |
|---|---|---|
| C Ionian (C Major) | C D E F G A B | CMaj7 |
| C Lydian (G Major) | C D E F# G A B | CMaj7 |
- Both these scales contain the notes C, E,G and B, so the chord CMaj7 can be created or derived out of both of these scales
- This means that both of these scales fit over the CMaj7 chord – so both can be used to improvise over a CMaj7 chord.
- And, in fact, the C Lydian Scale will fit better (that is, be more consonant) because it avoids the note F, which is an avoid note.
- So that means, when improvising you can use any scale over a chord, as long as that scale has all the notes found in the chord.
Axiom #4: If a scale contains all the notes in a particular chord, you can use that scale to improvise over that chord
- Now, it’s actually a bit more complex than this, but that’s the general idea
- So you could use the following scales to improvise over a II-V-I
| Chord | Dm7 | G7 | CMaj7 |
|---|---|---|---|
| Notes | D F A C | G B D F | C E G B |
| Scales | D Dorian | G Mixolydian | C Ionian |
| D Aeolian | G Wholetone | C Lydian | |
| D Blues Scale | G Lydian Dominant | C Lydian Augmented |
- So that’s the theory bit. Now for the practical bit.
Voicings
- Playing chord built up in 3rds (called block chords) is considered a bit simplistic
- So in Jazz we use more sophisticated ‘chord voicings’
- You play the same notes but in a different order and with differing intervals and across a number of octaves. As a general voicing rule:
- Use wide intervals at bottom; and narrower intervals at top
- And use Quartal voicings
- So you could play a CMaj13 chord as follows:
| Chord | Notes | Omission |
|---|---|---|
| CMaj13 | C E A B D; or C B D E A | Omits 5 & 11 |
| CMaj13 | B E A D G C | Omits 11 |
Improvisation
- Now in Jazz, you’re doing one of two things – you’re either in the rhythm section playing chords or you’re improvising – or both, with your left hand playing chords and your right hand improvising
- Jazz = Chords + Improvisation
Axiom #5: Improvisation is all about creating tension and then resolving that tension
- Creating tension = playing dissonant notes (avoid notes)
- Resolving tension = playing consonant notes (chord tones & available tensions)
- And there’s a million different ways to do that, but that’s just the underlying idea
How to Play Jazz Summary
- So that, in a nutshell, is Jazz Theory from the ground up.
- That was largely harmonic theory. I didn’t talk about meter or rhythm, which in Jazz is usually 4/4 and Swung.
- And I didn’t talk about form, which is usually a Head-Solo-Head form with the head being a 12 bar or 32 bar length.
- Playing Jazz requires playing chords and improvising. And it’s all about creating tension (with dissonance) and resolving that tension (with consonance) while doing those two things.
- Improvisation: Use notes from a scale that create dissonance and consonance based on the interval they imply with the ‘Tonal Centre’ and the underlying Chord
- Chords: Create dissonance and consonance by adding extensions and alterations to chords, through the chord progression and through voicings
Modern Jazz
- Everything I have explained here relates to ‘traditional’ ‘tonal’ Jazz – like Swing, Bebop, Hard-bop, and Cool Jazz
- More Modern Jazz – like Modal Jazz, Free Jazz, Post-Bop, and Contemporary Jazz – is all about breaking these rules by doing things like:
- Creating chords with avoid notes
- Creating non-functional chord progression
- Using non-diatonic scales
- Creating songs without chords or chord progressions
- But, of course, you have to know the rules in order to break them. And hopefully this Jazz Theory Overview gave you a nice quick refresher of what those rules are.
And on that note, let’s move on to Modern Jazz in our next lesson.